THE OBLIQUITY OF THE ECLIPTICAncient, mediaeval, and modern observations of the obliquity of the Ecliptic, measuring the inclination of the earth's axis, in ancient times and up to the present by George F. Dodwell B.A., FRAS*
George Dodwell was the government astronomer for South Australia. When he died in 1963, the Astronomical Society of South Australia requested Barry Setterfield to prepare Dodwell’s notes for publication. This Barry did. However he was unable to find a publisher in Australia for Dodwell’s works. A friend of his offered to take the material and have it published under the auspices of a university in the United States. That was in the late 1980’s. We were told of a number of different difficulties through the years which were delaying publication, but publication still has not happened. Recently we were sent some files of what this person had prepared and discovered he had made a number of typing errors and, at two points at least, had inserted his own sentences which were not in the original text and not marked them as separate from the original text. This is not acceptable. In the meantime, Dodwell’s sons were getting a bit impatient as well, and at their request and with their permission the material on the obliquity of the ecliptic (the tilt of the earth's axis) is now presented here in its entirety for the first time. Since we have the original manuscripts ourselves, every word has been checked and every punctuation mark, for accuracy. We have occasionally changed some paragraphing and punctuation to improve readability (Dodwell was very fond of commas), and have inserted some formal tables in place of the informal tables in the original manuscript. The figures have been scanned off the originals and sized as large as possible to permit reading of even the small type. The only major problem we encountered was a missing diagram of the Temple at Tiahuanaco (chapter 10) to which Dodwell refers in the last chapter. All emphases are in the original. So here, finally, is Dodwell’s work. We wish to extend a deep and sincere thanks to David Bowdoin for his hours of work in helping us get the old photographs and charts and diagrams from Dodwell prepared and ready to put here in the manuscript. We are also very grateful to John and Peter Dodwell for their help in locating the photographs on the photograph page, helping with the captions, and their encouragement throughout this process. We have added a number of other pictures in addition to Dodwell's originals which we think help the reader understand what is being written about. Sincerely, February 23, 2010 About George Dodwell (1879 - 1963) George Frederick Dodwell was born in Leighton Buzzard, England, on February 13th, 1879. After a move to Australia, Dodwell attained his degree at Adelaide University and became a Fellow of the Royal Astronomical Society. He was appointed to the staff of the Adelaide Observatory on March 1, 1899, as a Junior Computor (the person who was responsible for the mathematical calculations) under Sir Charles Todd who had himself been trained under Sir George Airy at the Royal Observatory, Greenwich. He succeeded Sir Charles, being appointed Government Astronomer for South Australia in June 1909. Dodwell held this position until his retirement on October 31, 1952. During his time at the Adelaide Observatory there were many achievements to his credit, about which he was typically humble. In 1900, shortly after the invention of wireless telegraphy, the Observatory became the site of the first wireless installation in Australia for distant signaling. The occasion arose to fix the boundary between South Australia and Western Australia, which was defined as the 129th Meridian East of Greenwich. It was George Dodwell himself who proposed that this be determined by wireless signals heard by Greenwich as well as the field stations at Deakin and Argyle Downs simultaneously. The radio time signals were transmitted from Bordeaux and Lyon and Annapolis. This international project linked the Greenwich, Paris and Washington Observatories as well as those in Australia from April to July 1921. It was the first time that the world had been measured from one side to the other by wireless telegraphy in conjunction with astronomical observation. This was a world “First” for both Adelaide and George Dodwell. Other work which brought the Adelaide Observatory world recognition under George Dodwell’s leadership included a magnetic survey of South Australia. This led on to his study of latitude variations which was held in conjunction with La Plata Observatory in Argentina, the International Latitude Congress and the International Astronomical Association. In September of 1922 he led a combined party with Sir Kerr Grant of Adelaide University to observe and photograph the total solar eclipse from Cordillo Downs, Australia, to test Einstein’s theory of the effect of gravitation on light. Also in that year he was appointed by the Commonwealth of Australia to be its representative at the International Astronomical conference in Rome. The study of latitude variations led George Dodwell to investigate the Obliquity of the Ecliptic (the tilt of the earth’s axis). This work commenced in 1934, a year after his uncle, Sir Frank Dyson, K.B.E., F.R.S., had retired as Astronomer Royal in England. An ancient manuscript by a Medieval Belgian astronomer Godefroid Wendelin, containing observations of the Obliquity, was published in 1933 and was obtained by Dodwell. The entire data set revealed a progressive and one-sided abnormality compared with Newcomb’s Formula. In 1935, Dodwell sent a preliminary paper to the Royal Astronomical Society. The referees suggested a further study of observational errors. This was undertaken experimentally at Adelaide University during June 1936 with nine experimenters making 172 observations on a vertical gnomon. An initial Report covering the period from December 1934 to December 1936 was made to the South Australian Parliament on February 20, 1937. On that occasion, Dodwell announced that an account was being prepared for publication. He wanted this research to be published as a Report of the Adelaide Observatory. However, the Observatory was closed in 1952 at his retirement. His research on the topic continued, but the Report was only completed just prior to his death on August 10, 1963. The following is the manuscript of his research.
********************************* Note: George Dodwell wrote two sections in his manuscript. The first had to do with biblical exposition. The second with the astronomical material. His astronomical research came first and his biblical application later in his life. This preface was for both sections and since we are only putting the astronomical material on our website, we have inserted ellipses in his preface where we have deleted references to his biblical material. This preface is the only place where any of his original material has been edited. Barry and Helen Setterfield AUTHOR'S PREFACEIn the year 1934 I had a great desire to do some particular work which might be of value in astronomy, and also be of general interest. … [Later], when looking through the library of the Adelaide Observatory, my attention was aroused by a book called “Draysonia,” written by the British Admiral Sir Algernon F. R. DeHorsey. It referred to an astronomical theory advanced by Major General Professor Alfred Wilks Drayson, who had been for 15 years a Professor at the Royal Military Academy at Woolwich, and also for two years an astronomer at the Royal Observatory, Greenwich. Admiral DeHorsey formerly had been one of his students at Woolwich, and had a great appreciation of his work.. The book gave an explanation of what Professor Drayson believed to be a movement of the earth, supplementary to the movements already known, and performed round what he called a “second pole of precession.” * He claimed that this movement accounted for the earth’s past geological climates and the ice ages. I was aware that Professor Drayson’s theory was regarded as a paradox, contrary to sound astronomical doctrine, by high astronomical authorities; and I wished to find out where it was in error. It soon became clear that the paradox lay in the fact that for this proposed physical and cyclical movement of the earth, which he believed to be still in progress, there was apparently no known or possible physical cause. Nevertheless, although the theory was thus untenable, it seemed to me worthwhile to trace out more clearly just how much, and why, the ancient and mediaeval observations of the obliquity of the ecliptic, on which Professor Drayson based his conclusions, differed from Newcomb’s internationally accepted formula for the secular, or age-long, variation of the obliquity. These observations went back to values given by Strabo, Proclus, Ptolemy, and Pappus in the early centuries of the Christian era. They indicated a consistent and increasing divergence in past ages from the values calculated by means of Newcomb’s formula. Sir George Airy, the seventh Astronomer Royal of England, long ago affirmed the principle, in his book on the Theory of Errors, that in astronomical and other statistical investigations, no observation, however apparently imperfect or unreliable by itself, should be rejected, but all observations, after correction for known sources of error, should be used in forming the average or mean result, since in a long series the very high values would tend to be counter-balanced by the very low ones. The validity of this principle is seen in an illustration, pointed out by A.R. Wallace, that the mean height of the tallest giant and the smallest dwarf in Great Britain is almost exactly the same as the average height of the whole population. It is also seen in target shooting, where, with a large number of shots, the mean position between the very high and very low shots, as also the very wide ones on both sides of the target, is found to be very close to the centre of the target. The correct principle, therefore, in studying the mediaeval and ancient observations of the obliquity of the ecliptic, should be, firstly, to obtain as many of these observations as possible; secondly, to correct each one, as far as possible, for any known or ascertainable source of error; and then to draw the curve which unites them all with one another and with the modern observations. Then, from the mathematical character of the curve, we may perhaps find some new truth or circumstance, disclosed by the observations, and previously unsuspected. This, accordingly, was the plan which I endeavoured to follow. I therefore wrote, and searched in numerous books, for this kind of information from ancient China, India, Egypt, and Greece, as well as from mediaeval Europe and parts of Asia. The results are given in Chapters 3 to 10, of this volume, Astronomical Investigations of the Obliquity of the Ecliptic. [note: this was his initial title which he himself changed later] At the beginning I had the good fortune to obtain a copy of the first manuscript of the famous mediaeval Belgian astronomer, Godefroid Wendelin, which had been lost for three centuries, until it was recently discovered in the Library of the City of Bruges. It was published in 1933 by M. l’Abbe G. Lemaitre, in Vol. 9. 1932 of the Publications of the Laboratory of Astronomy and Geodesy in the University of Louvain. This work contained, amongst other things, a list of ancient observations of the obliquity of the ecliptic, made by Thales, about 558 B.C.; Eratosthenes, about 230 B.C.; in his later years, Hipparchus, 135 B.C.; Ptolemy, 126 A.D.; and by several mediaeval astronomers up to the time of Tycho Brahe, 1587 A.D., and of Wendelin himself, 1616 A.D.; together with Wendelin’s theory of the cause of the change which had taken place during the ages up to his own time. My further searching for ancient and mediaeval observations of the obliquity of the ecliptic resulted in bringing together and analysing a very considerable and valuable series of these observations. The first curve of these ancient observations, which I drew in the year 1934, showed an unmistakable and progressive abnormality when compared with Newcomb’s formula. A careful study of the observations, and their agreement with the curve at all times and places of observation, showed that it was not due to large errors in the ancient observations. It was therefore very puzzling, as it seemed to indicate some unknown movement of the earth in the past. This could not be a cyclical or continuous periodic movement, for such an explanation would merely repeat the Draysonian paradox, and would be impossible. …After the initial drawing of the curve, uniting all the observations then available, I drew a subsidiary curve of “residuals,” that is, of the differences found by subtracting the quantities calculated by Newcomb’s formula from those given by the observations. Since Newcomb’s formula completely represents all the known forces governing the regular slow movement of the earth’s axis, a curve of residuals would tend to indicate some unknown condition. The residuals fitted closely on a mathematical curve, of a type with which I was not immediately familiar. I sought help in this difficulty from some learned mathematical friends, but without success. Finally, in Joseph Edwards’ Differential Calculus, p. 102, the curve I was seeking stood out unmistakably, demonstrating the principles of both “insensibility” and “irregularity.” It was a “logarithmic sine curve.” (see Fig. 3) When the scales of time and of degrees of displacement of the earth’s axis from the normal position were adjusted, so as to correspond with the scales in the example, the resulting mean curve of the observations was an exact replica of the particular logarithmic sine curve illustrated in the text book. The mean observational curve fitted exactly with this logarithmic sine curve, as “a hand in a glove.” The date of verticality of the curve, 2345 B.C… coincided with an “irregularity,” this being a large and sudden change in the inclination of the earth’s axis; and the date of “insensibility” or of equilibrium in the horizontal time scale of the curve, was 1850 A.D. It was absolutely certain that every point on the mean observational curve in Figure 3 is precisely a point on a logarithmic sine curve. The curve is therefore a certain and sure mathematical demonstration that in the year 2345 B.C., the earth’s axis was suddenly displaced by a major impact; and the curve shown in Figure 3 is a curve of the partial recovery of the earth to a state of equilibrium, at its normal inclination and conditions, as reached in 1850 A.D. It should be noted here that the dates corresponding to verticality and complete horizontality of the curve do not depend on any isolated or arbitrary selection of the observations, but they are determined by the complete contour of the curve, which conforms to a definite and precise mathematical pattern throughout the whole of its course. A close examination of the relationship of the observations to the mean curve next showed that they were arranged in groups alternately above and below the mean curve, with a period of about 600 years between groups. Solving this problem by what is called the “method of harmonic analysis,” I found this was indeed the case, and I was able to establish a formula satisfying these conditions. This formula, represented in graphic form in Figure 4, gives what is called a “harmonic sine curve with diminishing amplitude,” the amplitude, or departure above or below the mean position, being a maximum at the beginning of the curve, in 2345 B.C., and gradually being reduced to zero at its end, in 1850 A.D. This is characteristic of the way in which a freely spinning body, like the earth in its daily rotation, is restored to a steady condition after a sudden disturbance of its axis. The presence of this harmonic sine curve, with its diminishing amplitude, is a marvelous confirmation of the important, and now verified, fact that the earth has gradually been making a partial recovery, during the interval of 4194 years from 2345 B.C., to 1850 A.D., after a sudden large disturbance of its axis in 2345 B.C., and that it reached its present state of completed equilibrium in 1850 A.D. In 1935 I sent a preliminary paper to the Royal Astronomical Society, dealing with the results so far obtained at that time. It was returned to me by referees, with a note that the errors of the ancient observations needed further discussion. It was the general opinion of astronomers at that time, and still is, that the differences of the ancient observations of the obliquity of the ecliptic from Newcomb’s formula are wholly due to errors of the ancient observations. This opinion, however, is now shown to need revision, in the light of the examination of the question given in Chapter 2 of The Obliquity of the Ecliptic. The advice given at that time by the reviewers was most valuable, and I decided to examine the conditions of error in the ancient observations in a practical way, by constructing and using a type of vertical gnomon for solstitial observations of the sun, similar to the gnomons used in ancient times. This was thoroughly tested at the Adelaide University at the June (southern winter) solstice of the year 1936. Nine observers took part in the observations, and 172 observations were made. They are described in Chapter 2. The results confirm the general accuracy of the ancient observations, within one or two minutes of arc; and they show also that in a long series of such observations by a careful observer (such as the observations made by Eratosthenes during his 40 years work at Alexandria), no great errors occur. The next step was to write a full account of the whole matter, together with a study of the astronomy of the Solar Temples of Egypt, especially the Great Solar Temple of Amen-Ra at Karnak, which was oriented to the setting sun at the summer solstice at the date of its foundation, about 2045 B.C. To be studied along with this was the famous ancient British calendical monument of Stonehenge, which was oriented by its builders to the point of sunrise at the summer solstice in ancient times; and, in addition, the ancient great Peruvian Solar Temple of Tiahuanaca, near Lake Titicaca, in the Andes Mountains in Bolivia, designed by its builders for observation of the points of sunrise at both summer and winter solstices. All of these ancient solar temples or monuments throw a very interesting light on the obliquity of the ecliptic in ancient times, and are in full agreement with the results obtained from the ancient astronomical observations made by the Chinese, Hindus, and Greeks, as described in Chapters 3, 4, and 5. I hoped this work might be published as a product of the Adelaide Observatory, for distribution to other observatories, and for astronomers to examine. But its publication has been delayed.
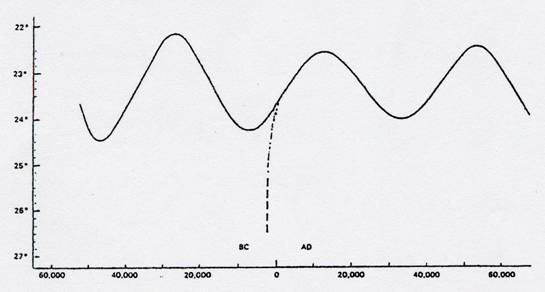
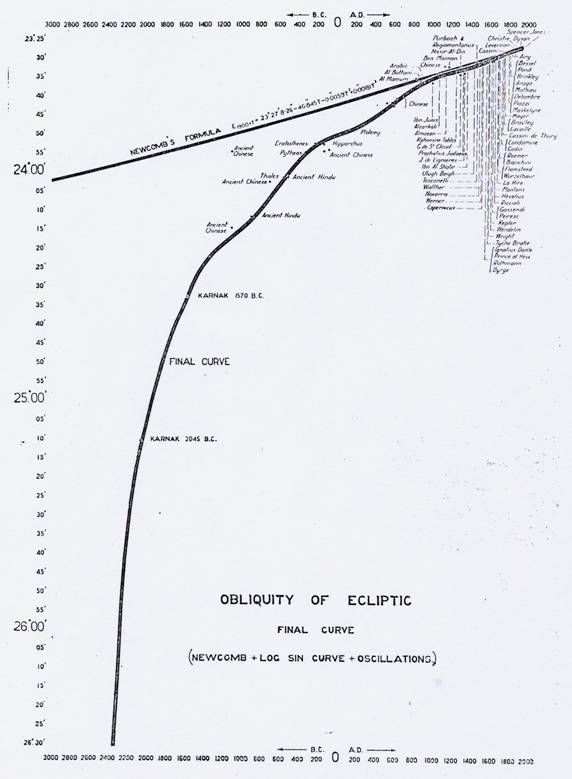
In this work, which has taken many years to complete, it is shown that the Ancient and Mediaeval Observations of the Obliquity of the Ecliptic (which is a measure of the Inclination of the Earth's Axis away from the perpendicular to its orbit), differ from the regular curve, corresponding to Newcomb's internationally accepted Formula, by an amount increasingly greater the farther we go back into antiquity. In Chapter 2 an account is given of the errors to which the ancient observations were liable, and of the corrections applied in order to obtain the true value at each date of observation. Chapters 3 to 7 give an account of the observations of the Obliquity of the Ecliptic made by the Ancient Chinese (3); Ancient Hindus (4); Ancient Greeks (5); the Mediaeval Arabs and Persians (6); and finally the European observations, both mediaeval and modern (7). Chapters 8 to 10 give an account of those famous monuments of antiquity, oriented to the sun at the Solstices, at Karnak and other places in Egypt, Stonehenge in England, and Tiahuanaco in Peru. THE MOVEMENT OF THE EARTH’S AXIS OF ROTATION IS EVIDENCE OF A Figure 1, below, is a graph of the regular, periodic variation of the Inclination of the Earth's Axis of Rotation, usually known as the secular, i.e., age-long, variation of the Obliquity of the Ecliptic. It is due to the gravitational attraction of the planets upon the Earth, interacting with the attraction of the Sun and Moon on the Earth's equatorial protuberance. In this graph the normal curve of the Obliquity of the Ecliptic is traced for three oscillations. The curve is derived from the comprehensive formula given by the American astronomer, J.N. Stockwell (Smithsonian Contributions to Knowledge, Vol. 18. 1873, Article 3). FIGURE 1
This formula, Stockwell says, enables us to obtain the numerical values of the Obliquity during all past and future ages. From the curve, we see that the obliquity, which is now (in 1954) 23º 26' 43", is gradually decreasing to a minimum of 22º 30' about 13,000 A.D.; and that it was at its last maximum, 24º 12', about 7000 B.C. The results obtained by Stockwell were slightly modified by the later researches of the American astronomer Simon Newcomb, whose formula, though more accurate for recent times, is not strictly applicable for more than 2000 or 3000 years before and after the present time. Newcomb's Formula has been adopted by astronomers as the International Formula for the secular, or age-long, variation of the Obliquity of the Ecliptic, within the limits of time just mentioned, that is, as far back as perhaps 1000 B.C. or 2000 B.C., and that which it will follow in similar ages to come. I have therefore used it here for all comparisons with ancient, mediaeval, and modern observations of the obliquity. It is the general belief of astronomers that Newcomb's Formula does truly and very closely represent the Obliquity, within the time limits mentioned; and the divergences of the Ancient and Mediaeval Observations from this Formula have been consequently ascribed to errors of these observations, due, it is generally thought, to the use of simple or crude instruments and methods of observation. Figure 1 shows, on a small scale, how the discrepancies appear when they are set out on a graph, and the Scale is magnified in Figure 2. The observations which they represent belong to widely separated times and places, the latter including Ancient China, India, Egypt, Greece, and various parts of Asia Minor and Europe. They agree so consistently, throughout all times and places, in tracing out a curve diverging more and more from Newcomb's Formula, as we go back to antiquity, that we must look carefully into them, to examine their reliability, and to see what light they throw upon the early state of the Inclination of the Earth's Axis. DISPLACEMENT OF THE EARTH’S AXIS. It will be seen that the curve, on which the actual observations fall, is a combination of
The mathematical interpretation of this second type of curve is that it is a curve of recovery after a disturbance of the Earth's Axis, the final state of equilibrium having been reached only in recent times, viz., about the middle of the 19th Century, or approximately 1850 A.D. That is why no trace of it is now revealed in the observations made with modern instruments of high precision, in the great Observatories of today.
FIGURE 2
See the Data Table for the list of observations The movement was still sufficiently in evidence, however, in the 17th Century, to be perceptible in the observations made by Flamsteed, (the first Astronomer Royal of England, at Greenwich Observatory), and in the observations also of other noted astronomers of that period. It is very much more noticeable in the earlier times. Nevertheless, in considering some of the better known ancient observations, such as those of Eratosthenes, 230 B.C., and Hipparchus, 135 B.C., (when the difference was as much as 8¢ greater than Newcomb's value), the confidence of astronomers in Newcomb's Formula is so strong, that they have only been able to conclude that these ancient observations are all erroneous, to the exact extent by which they differ from Newcomb.
Tannery also points out that Eratosthenes "naturally held to the process, already familiar in all the Greek cities, of observing the shadows of the gnomon.” (See, further, the detailed account of these observations made, over a period of many years, by Eratosthenes) With regard to Tannery's suggestion that the determination of 24 degrees for the Obliquity of the Ecliptic goes back to the School of Pythagoras, we may now see that this suggestion is correct. Pythagoras was born at Sidon in Syria about 580 B.C., or some years earlier. He was brought up at Samos, his father's principal residence. He commenced to travel in the pursuit of knowledge at the age of 18; at Miletus he conversed with Thales. He then traveled to Phoenicia and Syria, and then went to Egypt, where he spent 22 years in his studies, which were terminated by the conquest of Egypt by Cambyses in 525 B.C. In Egypt he studied especially geometry and astronomy, and extended the theorem of the "Square of the Hypotenuse" to all right-angled triangles, this theorem being associated ever since with his name. Jamblicus tells us that he practised astronomy in the Egyptian temples during the whole of his stay in Egypt. He was taken to Babylon as a captive, but continued his studies among the Chaldeans and the Persian magoi. After that he visited India, Celtica, Iberia and Sicily, and then settled at Crotona in Southern Italy. We may therefore conclude that the "School of Pythagoras," where he carried out astronomical observations, was established at Crotona about the year 515 B.C. From the New Formula, the Obliquity of the Ecliptic at this period was 24 degrees, 0 minutes, 5 seconds. Hence, Tannery was right in attributing the value of 24 degrees to a date corresponding to the School of Pythagoras. Let us now consider a little more closely this Obliquity-result of 24 degrees, associated with the name of Pythagoras. Pythagoras was a disciple of Thales, the first of the Seven Sages of Greece, who himself also made measurements of the Obliquity of the Ecliptic, and obtained the same result, 24 degrees. It may be considered certain that Thales used the vertical gnomon for these measurements, as he was a kinsman, companion, and fellow-townsman at Miletus of his famous younger contemporary Greek philosopher, Anaximander, who, having learnt the use of the vertical gnomon from the Chaldeans, is notable for being the first to introduce its use into Greece. With it he (Anaximander) observed the Obliquity of the Ecliptic. He also set up the first sun-dial in an open place at Lacedaemon. The vertical gnomon became known to the Greeks as a scioterion (“shadow-taker”), or heliotropion (“instrument for observing the sun at the solstices”). There can be no doubt that Pythagoras used this instrument at his school at Crotona, and with it observed the mid-day altitude of the sun at the summer and winter solstices. This was several centuries before the introduction of other types of astronomical instruments also used by the Greek astronomers of a later period at Alexandria. From these observations, Pythagoras would have obtained, (just as Eratosthenes did nearly three centuries afterwards), the double angle of obliquity, by subtracting the observed mid-day altitude of the sun, at the winter solstice, from its corresponding mid-day altitude at the summer solstice. This double angle of obliquity was 48 degrees, half of which, 24 degrees, is the Obliquity of the Ecliptic associated by Tannery with the name of Pythagoras. In this connection we may note that the ancient Greek astronomers sometimes give the double (or total inter-tropical) angle of Obliquity, as in the case of Thales (48 degrees), Eratosthenes (47 degrees, 42.5 minutes, whence the half part or the obliquity-- 23 degrees 51 minutes 15 seconds -- is derived), and Hipparchus (the same as Eratosthenes). In agreement with this, Ptolemy in his Syntaxis, states that the double angle of Obliquity observed by Eratosthenes and Hipparchus was less than 47 degrees 45 minutes, but greater than 47 degrees 40 minutes, (mean value 47 degrees 42.5 minutes), the half of which gives the Obliquity as 23 degrees, 51 minutes 15 seconds, as before stated. Sometimes, however, they give the ratio of this double angle to the whole circle of 360 degrees. Thus, Hipparchus gives the ratio of 11 to 83 for the total inter-tropical angle to the whole circle; this is equivalent to 47 degrees 42 minutes 39 seconds, from which is obtained the Obliquity 23 degrees 51 minutes 20 seconds, also attributed to Hipparchus. Another method used by the ancient Greeks was to give the ratio of the height of the gnomon to the length of the shadow at either or both solstices. As an example of this, Pytheas, the Ancient-Grecian explorer, on his voyage of discovery in 326 B.C., erected at Marseilles, at the summer solstice, a large gnomon, divided into 120 parts,* and gave the ratio of the height of the gnomon to the length of the shadow as 120 to 41 4/5 (according to Strabo), or (according to Ptolemy) as 60 to 20 5/6 or 120 to 41 3/5. The use of these large ratios, when a simpler ratio was not possible, indicated that the ancient Greek astronomers took considerable care with their observations, and did not merely give "round numbers."
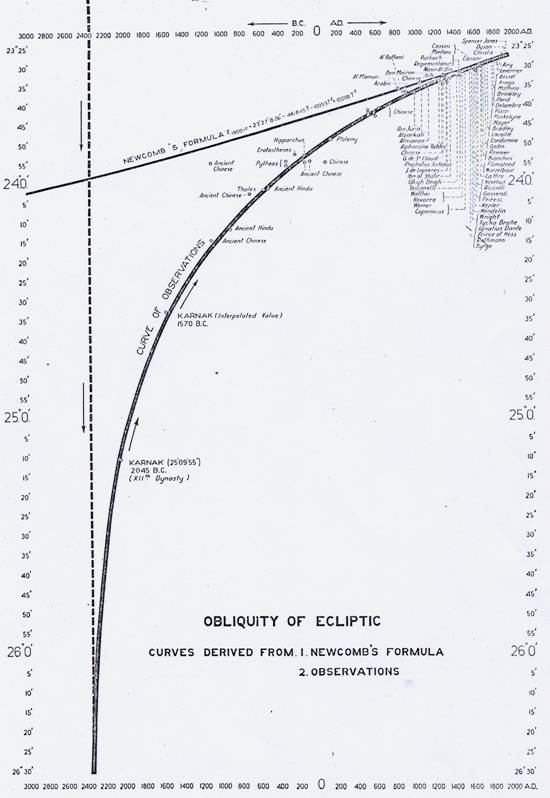
In the case of the observations believed to have been taken by Pythagoras at Crotona in Southern Italy, (latitude 39 degrees 7 minutes North), about 515 B.C., a simple calculation shows a glaring discrepancy in the shadow lengths of, let us say, an 8 foot gnomon at the winter solstice, according as we assume the Obliquity to be 23 degrees 45 minutes 30 seconds, given by Newcomb's Formula for that year, or 24 whole degrees, attributed to Pythagoras. For, in the former case, the winter shadow length would have been 15 feet 5.4 inches (Newcomb), whereas it would have been 15 feet 7.3 inches, i.e., a difference of 1.9 inches. We cannot think that Pythagoras could have ignored such a large difference. A similar criterion applies also to the observations made by Thales. Whatever the height of the gnomons used by them may have been, we may justly have confidence in the substantial accuracy of their observations. The observations of Thales and Pythagoras are also supported by the Hindu results, derived from the astronomy of the Surya Siddhanta, at about the same period; and the fact is that Newcomb's Formula differs from all the observations at this period by the surprisingly large amount of 15 minutes of arc, or 1/4 of a degree. Since Newcomb's Formula truly represents the factors governing the change in the obliquity as known to us, we can only conclude either that the ancient observations are greatly in error, or that there may have been some other factor causing a change, and not hitherto brought to light. In order to examine the accuracy of this explanation, I have commenced the account of the ancient observations with a study of the errors to which, in particular, the observations made with the vertical gnomon, or shadow pole, were liable, since this was the instrument most frequently used in ancient times for observations of the sun's altitude at the solstices. It was, in fact, regularly used for such observations in China for a period of more than 2000 years, as far back as 1100 B.C. So important was it considered by the Chinese that uniformity of observations should be established, that they fixed by law the height of the gnomon at 8 feet. The gnomon was also used by the ancient Hindus and by the Greeks. At a later date, from the time of Eratosthenes, 230 B.C., the Greek astronomers introduced armillae and other circular instruments; but the gnomon still remained in general use, and Ptolemy and Strabo regularly record latitudes in terms of the height of the gnomon to the length of its shadow. On the revival of astronomy in the 9th Century A.D., the Arabs and other mediaeval astronomers also used the gnomon for observations of the sun's altitude, and it was the standard meridian instrument at the great Observatories of Paris and St. Petersburg as late as the middle of the 18th century. The results obtained with the gnomon are so well within the limits of error required for the present investigation that the observations made with it in ancient times are worthy of close attention. They show that the large divergences from Newcomb's Formula are not simply due to errors of observation, but indicate another factor, which calls for explanation. After due allowance is made for all sources of error, the mediaeval and ancient observations of the obliquity are found to lie consistently on a curve, shown in Figure 2, which is in agreement with Newcomb's Formula in modern times, but differs greatly from it 2000 or 3000 years ago. CONFIRMATORY EVIDENCE A remarkable confirmation of the obliquity in the year 2045 B.C. is given by the oriented solar Temple of Amen Ra at Karnak, Egypt, the largest temple that has ever been built. This is described in Chapter 8. Hieroglyphic inscriptions, engraved in granite, show that the ancient Egyptians took great pains to orient their solar temples. The rays of the rising or setting sun, on certain important dates, then shone straight down the long central avenue, or temple axis, into the darkened sanctuary and illuminated the image of the sun-god within it. In the great Solar Temple at Karnak, this occurred at sunset on the day of the summer solstice. It will be shown that in 2045 B.C., the foundation date of the Temple, the sun's solstitial declination, or maximum distance from the celestial equator, was 25 degrees, 9 minutes, 55 seconds. This value is in exact agreement with the prolongation of the curve of the ancient observations, and exceeds Newcomb's value for that date by 1 degree 14 minutes. Further confirmation, at other points on the curve, is given by the famous solar monuments at Stonehenge, England, and Tiahuanaco, Peru. COMPARISON OF NEWCOMB'S FORMULA WITH THE ANCIENT OBSERVATIONS We must now consider the relationship between the two curves. This is shown graphically in Figures 1 and 2.
(b) The range of the obliquity
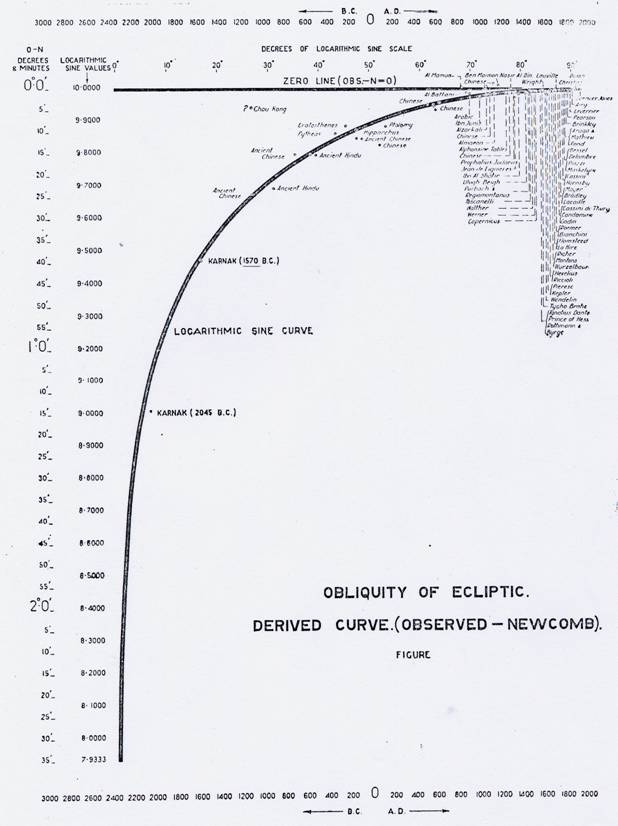
Figure 2 shows the relationship of the curve of the observations to that of Newcomb's Formula back to 3000 B.C., on a larger scale than in Figure 1, and with more detail. THE NEW CURVE OF OBLIQUITY FOR PAST AGES To find out what that is, the sun's solstitial position or declination, north or south of the celestial equator, is taken as the normal one for any given date, and is subtracted from the observed declination on that date. We then get a collection of differences, or "residuals," which lie upon, or close to, the smooth curve shown in Figure 3. FIGURE 3
THE CURVE OF RESIDUALS It will be seen by inspection that when the curve is produced downwards, it tends to become vertical about the date 2345 B.C. Continuing the curve upwards, it becomes completely horizontal about the date 1850 A.D. This curve is a logarithmic one. It corresponds exactly to a curve of “logarithmic sines." The reader who is well acquainted with mathematical curves can at once recognize this by inspection. It may also be shown in a graphical way (see Fig. 3). To do this we write beneath the date scale of B.C. and A.D. centuries, in the top horizontal line, a scale of degrees from 0º to 90º. The beginning of this degree scale, 0, is at 2345 B.C., where the curve is vertical; and the end of the degree scale, 90º, is at 1850 A.D., where the curve is completely horizontal. The full period covered by the curve is thus 4194 years, and each degree of the scale corresponds to 46.6 years. We now construct a vertical column (Column 2) of the numerical values of logarithmic sines, from 90º to 0º, taken from a book of logarithms. The starting point of this column, on the left hand side of the "Zero Line," is the logarithmic sine of 90 degrees, namely 10.0000. It refers to a final point on the curve at 1850 A.D., where the curve ends horizontally. The numbers given in Column 2 and the degrees and minutes of which they are the logarithmic sines, are shown in the following table. TABLE I
A simple scale relationship can be seen to exist between the numbers in Column 2 and those in Column 1 in Figure 3 (i.e., the residuals or differences between Newcomb and observed obliquities), namely a difference of 0.1000 in the numbers in Column 2 is equivalent to a difference of 7.5' in Column 1. This enables the numbers in Column 2 to be set down in their exact position on the graph. The relationship of the horizontal and vertical co-ordinates is then such that, if pairs of lines, horizontal and vertical, are drawn on the graph from points indicated by the table above, each pair will meet exactly on the observational curve. Thus, they all agree completely in showing that the curve which fits the ancient, mediaeval, and recent observations between 2345 B.C., and 1850 A.D., is none other than an exact logarithmic sine curve. This type of curve is illustrated in J. Edwards' Differential Calculus 1896, p. 102. It is a curve of recovery, with restoration to equilibrium after a disturbance; and it shows with certainty that a disturbance of the earth's axis occurred at the date 2345 B.C., corresponding to the 0º end of the curve; and that its restoration to equilibrium was completed by the year 1850 A.D., corresponding to the 90º end of the curve. The formula is:
This formula enables us to calculate the Mean Curve value for any date between 2345 B.C., and 1850 A.D.
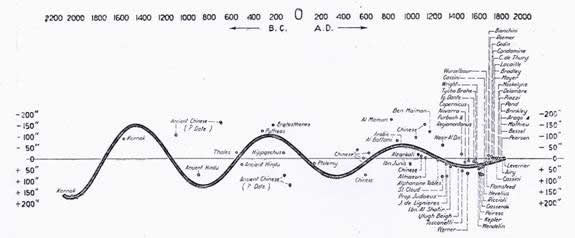
In a logarithmic sine curve, the numerical value at 0º is infinite. Therefore, just at the beginning this curve fails to represent the phenomenon as it actually happens, since it has a finite commencement. Nevertheless, soon after this initial irregularity, the curve representing the phenomenon links on normally to the logarithmic sine curve, and continues thus to the end. From a study of the starting point of other natural phenomena, which accord with a logarithmic sine curve (e.g. the first or major phase of the light curve of a new star after its initial outburst), it seems probable that the earth's rotational axis was suddenly changed by the force of impact in 2345 B.C., from an original inclination of about 5 degrees, by an amount of about 21.5 degrees to a new inclination of about 26.5 degrees. The vast movements and changes which took place at the time of the impact, and which were followed by continuing after-effects during later centuries, cannot easily be imagined, owing to the magnitude of the forces in operation, involving both the external and internal state of the earth. We may note, however, the Hebrew traditions about some of them , and the statements of Halley and Laplace. OSCILLATIONS A further unique circumstance, confirming the accuracy of the foregoing conclusions, is seen in the analysis of the differences of the individual results from the mean logarithmic sine curve shown in Figure 3. In accordance with the law of errors, they are distributed fairly evenly above and below the Mean Curve. But on closer examination, they appear to cluster in groups sometimes slightly above, and sometimes slightly below, the Mean Curve; the time interval between groups, from the highest to the lowest values, is about 600 years. They may therefore be examined by the method of harmonic analysis. As a first step they are plotted on a graph (Figure 4) and it will be seen at once that they fall upon a subsidiary curve of oscillations. It is important to notice that they are of diminishing amplitude, being much greater at the beginning than at the end, in 1850 A.D., where they become zero.
Seven semi-oscillations are indicated between 2345 B.C. and 1850 A.D., the exact semi-oscillation period being 599 years. The nodal points are at B.C., 2345, 1746, 1147, 548, and A.D., 52, 651, 1250, 1849-1850. The maxima and minima are alternately at the intermediate dates, B.C., 2045, 1446, 847, 248; A.D., 352, 951 and 1550. Also, when limiting lines are drawn through the maxima, and through the minima, they converge towards the zero point at 1850 A.D., where the principal or Mean Curve also is finished. This date is within the epoch of modern exact astronomical observations; so that there is nothing left of either the Mean Curve or the oscillations to affect modern observations. Hence this movement has not come to the notice of astronomical observers in the last 100 years.
These oscillations, in a magnified form, are shown in Figure 4. They point to an inter-action of the earlier precessional forces, affecting the earth's axis in its former nearly upright position [see note from Barry Setterfield], with those later predominating ones associated with its new and greater inclination. The curve of oscillations may thus be regarded as the resultant of these forces, the latter tending to retard, and the former to hurry the precession. The curve, which is a harmonic sine curve, with diminishing amplitudes, is in harmony with the consequences of the disturbance of a spinning body. The oscillations are accounted for by the inertia of the rotating body, alternately retarding and hurrying the precession (4). The rule is stated thus:
It is obvious that the immense mass of the earth, and its internal viscosity, play an important part in determining the magnitude and period of these oscillations. On account of its rotation, the earth may be regarded as a gyrostat on a very great scale; and what happens in the case of a disturbance is illustrated by the Brennan mono-rail car, which is balanced by a gyrostat. When the disturbance, (in the case of the Brennan mono-rail car, sudden wind pressure), is applied, the car heels over into the wind, (the direction of tilt being reversed by a special device). The final tilted position is reached with a certain momentum, which carries the car beyond the neutral position. The car over-shoots the mark, and returns, with oscillations of diminishing amplitude, until the steady point is reached. (6) The analogy with the earth's return to a steady point in 1850 A.D., during the period of 4194 years after its axial disturbance in 2345 B.C., is evident. The oscillations, thus disclosed by the harmonic analysis of the observations, are a striking confirmation of the disturbance itself, and of the course of the earth's recovery in the subsequent ages. FINAL CURVE OF OBLIQUITY. FIGURE 5
INTERPRETATION OF THE CURVE In considering the meaning of the Logarithmic Sine Curve, the question arises whether it is the only curve which could be drawn to fit the observations. It has been suggested that perhaps they might agree with some form of sine curve, indicating an unknown periodic movement of the earth's axis of rotation. The periodic movements of the earth's axis, however, are completely known; and there is no force available to cause any additional movement. The principal movements of the earth's axis of rotation, in order of their discovery are:
What is known as the "general precession" combines the luni-solar and planetary precessions. The luni-solar precession is a gyratory movement (like the wobble of a spinning top) of the earth's axis round a central point, the pole of the ecliptic, in an average period of 25,700 years, this period being lengthened to 25,800 years by the effect of planetary precession. The earth's precessional movement was one of the great discoveries of Hipparchus, about 124 B.C. A change in the obliquity of the ecliptic, dependent on the planetary precession, was first suspected by Eratosthenes in 230 B.C. As we have seen above, Eratosthenes found, from a long series of observations, that the obliquity was 23º 52' in his time, so that it was 8' less than the value 24º handed down from the time of Thales, in 558 B.C., and of Pythagoras, in 515 B.C.; i.e. a decrease of 8' in about 300 years. Nearly 2.5 centuries later, about 14 A.D., at the end of the reign of the Emperor Augustus, the Roman mathematician Manilius noted a change in the obliquity of the ecliptic, which he attributed either, as he says, to "the discordant course of the sun itself, and some change in the sky, or through some change in the universal earth, by which it has moved away from its center, as I have detected myself, and I hear of also in other places." This remarkable conclusion was reached by Manilius from his observations, during 30 years, of the solar shadows at the summer and winter solstices, cast by the great obelisk in Rome. This great obelisk, 75 feet high, was provided by Manilius with a golden ball at its summit, and the position of the circular shadow on the flat pavement below was carefully measured by him, over a period of 30 years, with a brass scale fixed into the pavement.(7) This movement of the earth, together with a supposed backward and forward movement of the equinoctial points, came to be associated in later years with theories of "trepidation," which was defined as "a motion ascribed to the firmament, to account for certain small changes in the position of the ecliptic and the stars." Copernicus, in 1525 A.D., and Wendelin, the noted Belgian astronomer, nearly 100 years later, endeavoured to explain the precession of the equinoxes, and the variation in the obliquity of the ecliptic, by trepidational theories. These were not on a sound basis, however, and no physical principle could be found to account for the movements. The precession of the equinoxes was, in fact, inexplicable by astronomers for 1800 years, from the time of Hipparchus until the great discovery of universal attraction by Sir Isaac Newton. In Newton's Principia, Book 3, Proposition 39, the problem of the earth's precessional movement was, for the first time, correctly explained and calculated. It was shown to be due to the gravitational attraction of the sun and moon on the earth's equatorial protuberance. Two hundred years after Newton, Sir George Airy, the seventh Astronomer Royal of England, said that "if we might presume to select the part of the Principia which probably astonished and delighted and satisfied its readers more than any other, we should fix without hesitation on the explanation of the precession of the equinoxes," Newton's Principia was published in 1697; and 60 years afterwards, Bradley the third Astronomer Royal of England, completed his famous series of observations of zenith stars, lasting for a complete 19 year lunar cycle. This resulted in his discovery of "nutation," a periodical slight change in the rate of precession, accompanied by a 9.5- yearly change of 9" in the inclination of the earth's axis, on each side of its mean position. The complete period was thus 19 years. Bradley's discovery was published in 1748, and in explaining it, he indicated the true cause, that the "nutation" or nodding of the earth's axis, was due to the variability of the moon's action on the earth's equatorial protuberance, on account of the moon moving in an orbit inclined 5º 9' to the plane of the Ecliptic. In the following year the eminent French mathematician D'Alembert published a treatise, in which a rigorous mathematical analysis conclusively proved this to be correct, and, in addition, it gave complete confirmation of Newton's explanation of precession. In Newton's time the gradual diminution of the Obliquity of the Ecliptic had not been fully established. Some astronomers, like Tycho Brahe, Riccioli, Gassendi and Flamsteed, believed that the discrepancies, from ancient times until their own, were due to errors of observation. Halley, the second Astronomer Royal of England, also held this opinion. In the year in which Newton's Principia was published, 1697, Halley presented a paper to the Royal Society, comparing the solstitial altitudes of the sun observed at Nuremberg by Wurtzelbaur in 1686 with similar observations made at the same town by Bernard Walther 200 years earlier. In this paper he said: "from these observations it appears that the obliquity of the ecliptic has continued unaltered for these 200 years last past, that is to say, that the angle which the earth's axis makes with the plane of the Ecliptic, or orbit wherein she moves annually around the sun, has been without sensible change in all that time.” Newton, however, had shown in the Principia, in general terms, that the perturbing effect of the planets would produce, among other things, an alteration in the inclination of the plane in which any one planet moves. He did not make any calculations of the amount of this change, but in this he indicated the cause which, in the case of the earth, produces the secular variation of the obliquity of the Ecliptic. The question of planetary perturbations thus began to attract the attention of astronomers, and in 1756 the Swiss mathematician, Euler, in a series of papers, showed that the effect of these perturbations on the earth would be not only to cause a slight movement of the precession in the opposite direction to that produced by the sun and the moon, but also to cause the obliquity of the Ecliptic to diminish about 48" in 100 years; not much more than 1" higher than the true value. Nearly 30 years later, the great mathematician Lagrange showed that the Obliquity could not diminish indefinitely, but that the action of the planets could only cause small oscillations in the positions of the various orbits, so that, in the case of the earth, the obliquity of the Ecliptic was confined within small limits. Laplace, in 1827, calculated the total range of this oscillation of the earth's orbit to be 3º 7' 30"; but a later calculation by Stockwell in 1873, using more accurate determinations of the masses of the planets, made the total range somewhat less, namely, 2º 37' 22"; the limits of the obliquity ranging from a minimum of 21º 58' 36" to a maximum of 24º 35' 38". Laplace, in The System of the World, Vol. 2. p. 211, explained the variation in these words:
Stockwell also commented in a similar way on the inter-action of the sun, moon and planets, in producing the variation of the obliquity, as follows:
Other eminent mathematicians, from the time of Euler, also made contributions to the theory of planetary perturbations, and of the precessional movement; so that, before the end of the nineteenth century, the action of the sun, moon and planets upon the earth's axis of rotation became fully understood and accurately calculated. To sum up, therefore, the external forces, acting upon the earth, and affecting its axis of rotation, are the gravitational attractions of the sun, moon and planets. Of these, the sun and moon cause the luni-solar precession, consisting of a very slow gyratory movement of the earth's axis round the pole of the Ecliptic as center, in an average period of 25,700 years, lengthened by planetary precession to 25,800 years. The inclination of the axis, however, is maintained at an almost constant angle so far as changes of a relatively short period are concerned; the principal variation of this kind, viz., that of the 19-year lunar nutation, being only 9" on each side of the mean path of precession. The attractions of the planets, combined with the sun and moon, however, cause a displacement in the plane of the Ecliptic, so that it oscillates with respect to the equator in a long period, varying from 26,000 years to 53,000 years, and this produces in the obliquity a series of maximum and minimum, having a total variation of 2º 37' 22", namely, from an absolute maximum of 24º 35' 38" down to an absolute minimum of 21º 58' 36", according to Stockwell's calculations. There are no other external forces acting on the earth to produce any additional change in the obliquity of the Ecliptic; but the question may still be asked whether there are internal conditions in the earth itself which may affect its movement. If there were an irregularity in the internal distribution of the earth's mass, causing a displacement of the center of gravity from the exact center of the earth's figure, would this produce any change in the obliquity? In such a case, the "axis of figure" of the earth would not correspond exactly with the earth's "axis of revolution," and there could be an inclination of one to the other. An oscillation would then be produced, but it would not be an oscillation of the obliquity, but one of a different kind, viz., an "Eulerian Nutation." This is a bodily oscillation of the earth's figure around the axis of rotation, which remains fixed in space, so that the position of the celestial pole, (i.e., the point in the sky marking the prolongation of the earth's axis of rotation) remains unaltered; in other words, the obliquity of the Ecliptic is not affected in the smallest degree by the "Eulerian Nutation." There is, in fact, such a movement of the earth, an "Eulerian Nutation," but of an exceedingly small amount. It is apparent in astronomical observations as a "variation of latitude." The first exact proof of its existence was obtained in the year 1888, and since 1900 it has been continuously observed at a number of international latitude stations, especially chosen for the purpose. This variation is irregular, and so small that it is difficult to observe, being always less than 0".5 from the mean value. This represents a movement of the terrestrial pole less than 50 feet from its mean position; and there is no evidence of any large change of latitude at the earth's surface during historical times. The possibility of a larger variation has been considered, and it has been shown that, if by any means a considerable deviation were effected of the earth’s axis of figure from the axis of rotation, then a large variation of latitude would occur. This variation would have a period of 305 days if the earth were perfectly rigid, but 432 days under its present condition of elasticity -- this being the period derived from the international latitude observations. From this lengthened period it has been concluded that the earth, though not perfectly rigid, is yet as rigid as steel. Further, it has been shown by Professor J.F. Twisden, in 1878, that if the latitude variation were of large dimensions, “enormous tidal motions of the ocean would be produced, and its waters would sweep over the continents much as a rising tide sweeps over a low bank on a level shore.” (J.F. Twisden. “On Possible Displacements of the Earth’s Axis of Figure produced by Elevations and Depressions of her Surface,” Quarterly Journal of the Geological Society of London, Vol. 34, 1878, p. 35 etc.) Such a tide does not exist, and the only observed tidal oscillation, having a period of about 430 days, is so minute that it is not certain whether a very slight effect of this kind, apparently observed by Bakhuysen in Holland and Christie in U.S.A., was due to accidental agreement or not. In addition to the tidal observations, those of Latitude Variation, as well as of Geodesy and Seismology, all agree in showing that the earth’s material is so symmetrically disposed with regard to the earth’s centre of gravity, and its rotational axis, that no large Eulerian Nutation is possible. Further, as indicated above, such a nutation, even if it did exist, would not be accompanied by any change in the Obliquity of the Ecliptic. We may say, therefore, with certainty, that the variation of the Obliquity of the Ecliptic, under the action of all the forces now at work, is completely known, and has been calculated, with the greatest precision; so that we are obliged to reject any suggestion that the curve of residuals in Figure 3 corresponds to some unknown periodic movement of the earth. The conclusion thus reached is that the deviation from the theoretical curve of obliquity is due either to errors of observation, or to the existence of some abnormality of an unexpected kind. The following pages show that errors of observation are quite inadequate to explain the increasingly large deviation when the curve is traced back to ancient times. Also, since the curve itself is so plainly a logarithmic one, we are limited to the interpretation which that implies. That is to say, at the zero end, where the curve becomes vertical there is “irregularity,” corresponding to a sudden and major disturbance of the earth’s axis; and at the 90º end, where the curve becomes horizontal, there is “insensibility,” or restoration to equilibrium. In other words, it is a curve of recovery after a large disturbance of the earth’s axis of rotation, the disturbance having occurred in the year 2345 B.C., and the restoration to equilibrium having been brought to completion in the year 1850 A.D. ********************* 1. Connaissance des Temps. 1827, p. 234. return to text ********************** note from Barry Setterfield: Dodwell has assumed here that the original axis tilt of the earth, before 2345 B.C., was nearly upright. For that reason, he supposed a very strong impact was necessary to jolt the earth from that position to its current 23.5 degree tilt. This is why a number of astronomers have rejected Dodwell’s work in this area. However, if the axis tilt was greater than its current axis tilt before 2345 B.C., then an impact of much less force would have been required to restore the earth to a slightly more upright position. The evidence for this greater axis tilt may be seen in the evidence of the ice age which covered most of Europe prior to 2345 B.C. Work by Dr. Benny Pieser of the Cambridge Conference Group and Dr. Moe Mandelkehr have shown that in period around 2345 B.C. climate, geological and archaeological changes occurred in which some important civilizations were destroyed -- it appears the first Intermediate Period in Egypt occurred at this time. See the Conclusion for a list of references. Return to text. |