TECHNICAL APPENDIX THE ZPE-PLASMA MODEL AND QUANTIZED REDSHIFTS. It has been pointed out that there seems to be a mathematical inconsistency in the formulation of the redshift equations in Quantized Redshifts and the Zero Point Energy; that is equations (18) to (26) in that paper. In order to discern what is happening with the redshift and a changing Zero Point Energy (ZPE), let us start with Puthoff’s 1987 paper where the ZPE effects on the atom are introduced. Puthoff pointed out that, for an electron to maintain its stable motion around a nucleus, the power it absorbs from the Zero Point Energy (ZPE) must be equal to the power it radiates as a result of its motion. Puthoff derived an equation for the power absorbed, and one for the power that was radiated. For stable, predictable motion, these two equations must be equal to each other. When this is done, the end result is Bohr’s equation for a stable orbit, namely that
In equation (A), h is Planck’s constant, which is a measure of the strength of the ZPE, m is the electron rest-mass, a is the orbit radius, and v is the orbital velocity. In a ZPE context, this means that the power transfer from the ZPE to electrons in their atomic configuration is accomplished through their angular momentum. However, both the September 2007 and the December 2008 papers show that the strength of the ZPE is increasing and the reason why is elucidated there. These papers show that an increasing ZPE strength with time inevitably means that both h and m must also increase with time. But in (A), the quantity mva represents the angular momentum of the electron in its orbit. Therefore, an increasing h means that the orbital angular momentum is also increasing as the ZPE strength increases. As the angular momentum changes, there must be changes in the orbit radius to compensate. Let us assume that orbit radii can only change in jumps of whole multiples of h. This is basically the same as Bohr’s original proposal that dealt with changes in angular momentum in atomic orbits. If we now designate those whole multiples by the letter N, so N = 1, 2, 3 …etc., then this condition means the orbit radius can only change such that a is proportional to 1/(Nh). However, h is smoothly increasing with time as the ZPE is strengthening. Therefore, we need to choose a value of h which is fixed and unchanging for the jump in N to be referred to. For the moment we will simply label this fixed value of Planck’s constant as ho. We can now write the expression for the orbit radius a as:
In equation (B), the symbol (~) means “proportional to”. This equation means that orbit radii remain fixed as time increases until a whole multiple of ho has been reached. At that time N will jump to the next higher integer. This is called a quantum jump. The period between such jumps is called the interval.
We can then substitute the results from (B) and (C.) back into equation (A). When we do this we find that, in order for equation (A) to balance, the rest-mass of the electron must obey the following proportionality
This is in accord with the observational data which has shown that sub-atomic masses are proportional to 1/c2 or proportional to h2 [see Reviewing the Zero Point Energy and its references]. In equation (D) it can be seen that, in the interval, N remains fixed so that, in the interval, m is indeed proportional to h2. However, as time goes on, and the Zero Point Energy strengthens, a moment is reached when h has just exceeded the next integer value of ho and N goes to its next value. At that precise moment, there has been an infinitesimally small increase in h, so for all practical purposes we can say that, at the exact moment of the quantum jump, h remains constant. But at that precise moment, N has increased by one, so there is a small quantum increase in m. The physical reason why the electron rest mass increases at the jump can be found in the nature of the electron itself. Puthoff has shown [in his 11th May 2007 paper in the International Journal of Theoretical Physics, vol 46, pp.3005-3008] that there is a pressure within an electron which comes from its electronic charge which tends to expand its radius. However this is offset by the external pressure of the ZPE which keeps it intact. The equations show that, if the ZPE strength increases, there will be a moment when the external pressure overcomes the internal pressure and the actual electron point radius undergoes a quantum decrease which effectively gives a quantum increase in its mass. There is a reason why there is a mass increase as the electron size reduces. As noted in Reviewing the Zero Point Energy, mass originates with the jiggling of the electron by the impacting waves of the ZPE. As the ZPE strength increases smoothly, so, too, do the number of waves of a given wavelength hitting the electron per second. This causes its jiggle volume to smoothly increase. The increased jiggling results in the electron taking up a larger volume. This increased energy of motion appears as an increase in mass. The quantum jump occurs at the moment when the internal pressure of the electronic charge can no longer resist the increasing Zero Point Energy. At that moment, the ZPE does not make any sudden change, but the 'straw that broke the camel's back' had just occurred and the electron finally reacts. The electron’s actual size has decreased (as distinct from its jiggle volume), and the electron will now resonate or jiggle to waves of a smaller wavelength. Because there are more ZPE waves of smaller wavelengths than longer wavelengths, this reduction in the actual electron size means that the greater numbers of smaller waves will cause the electron to jiggle more violently. This results in an increase in jiggle volume and mass. Let us now return to our redshift analysis. To check the equations so far, the proportionalities in the quantities in (C.) and (D) can be inserted into either equation (4-12) or (4-15) for electron orbit radii as given by M. R. Wehr and J. A. Richards in Physics of the Atom (Addison-Wesley Publishing Company, Reading, Massachusetts, USA, 1960), pages 86 and 87. These equations balance if the orbit radius has a 1/Nho proportionality as in (B). Furthermore, Bohr’s second equation which states that
will also balance with these proportionalities. In equation (E), the quantity e is the electronic charge and ε is the electric permittivity of free space. In the ZPE scenario, the term e2/ε is invariant, with the possible exception of strong gravitational fields. This invariance is upheld when (B), (C.), and (D) are substituted into (E). Let us summarize the analysis to this point and show how this results in a redshift. As time progresses and the strength of the ZPE builds up, atomic orbits must adjust to the increased power available to them from the vacuum coupled with increased atomic masses. To offset these two changes, the atom adjusts by making a quantum change in the radii of atomic orbits. Thus, as time moves forward, the radius of each orbit decreases in a series of steps. Each step has the effect of making the orbits further apart. Calculation shows that this occurs because the outermost orbit has essentially no change in its radius, or at most a very minor change, while the innermost orbit moves the most. All other orbits move inwards in proportion.
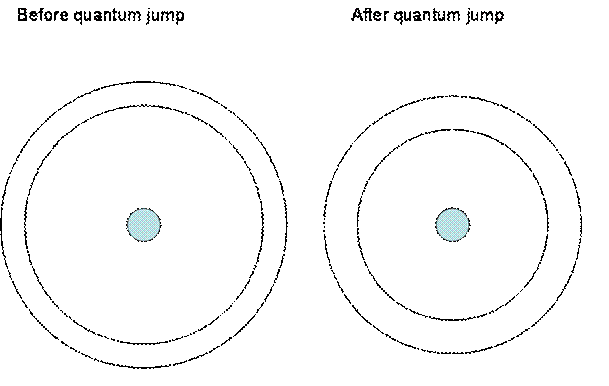
This diagram shows the inner two orbits of electrons in the elements involved, while the outer electrons are remaining at essentially the same distance from the nuclei. When an outside force excites an electron, forcing it out of its normal position relative to the nucleus, it will release the energy from that force as a photon of light when it snaps back into position. However, because the orbits are now further apart, there is a greater energy difference between them. This means the electron requires more energy to be forced into another position and thus will release more energy when it snaps back. The wavelength of light emitted from atoms depends on the energy difference between an outer orbit and an inner orbit as an electron 'falls' from one to the other. The greater the energy difference, the more energetic the photon, and thus the shorter the wavelength and the bluer the light that the electron emits. During the interval between quantum jumps, all orbit positions remain fixed, and so light emitted during that time will have a fixed wavelength. But, as time goes on, a quantum jump occurs, and all orbit radii then become less. The emitted light then becomes more energetic, not smoothly, but in a jump, so the wavelength of that light becomes shorter or bluer in a jump. This is why the redshift measurements are quantized. As we look farther out in space, and thus further back in time, we see that the light was LESS energetic before, and thus it appears red shifted compared to today's standard. The actual point is not so much that the light is 'red shifted' as we look back in time, but that is has become 'blue shifted' and more energetic (in jumps) as we approach our present time. This is a good time to remind the reader that the quantized red shifting is not seen inside of our local group of galaxies for the reasons given in the main paper. The quantized red shifts can be discussed in terms of physics. It needs to hold up mathematically. The equation for the energy of a given orbit can be written (as given by Wehr and Richards, op. cit. p.88 equation 4-18):
In equation (F), the radius of the orbit under discussion, a, appears in the denominator. Since the ratio e2/ε is invariant with time, then substituting the result from (B) into (F) gives us the proportionality
Therefore, as N increases with time, the magnitude of the orbit energy also increases. Electromagnetic radiation, including visible light, is emitted when an electron falls from an outer orbit to an inner orbit. The energy of the light emitted is equal to the difference in energy between the two orbits. If the outer orbit quantum number is given by n2 and the inner orbit by n1, then it can be shown that the energy emitted by the electron’s transition from the outer to the inner orbit has a wavelength given by λ such that
Now it has been shown in Reviewing the Zero Point Energy that the quantity hc is invariant throughout the universe. Therefore, substitution of the results from (D) into (H) gives us the information that for any given electron transition
In other words, as time moves forwards and the number of quantum transitions (given by N) increases, wavelengths, λ , become shorter in steps of 1/N. We can now substitute the results from equation (J) into the standard redshift formula, which states that the redshift, z, is given by
In equation (K), λ2 is our standard laboratory wavelength of a specific spectral line from an atom, while λ1 is the wavelength of the same spectral line emitted from a distant galaxy. But according to the approach adopted here, the wavelength of light emitted by a distant galaxy is only dependent upon the value of N which pertained then. Therefore we can substitute the results of (J) into (K) to obtain the outcome that
This therefore means that
where N1 is the value of N that was current at the time the light was emitted from a distant galaxy, and N2 is the value of N that we have at our present epoch. Since N represents the number of integer changes in ho, the value of z, the redshift, also allows us to determine the ratio, N2/N1, as in (M). Thus, by way of illustration, if we take N2 to have a value of, say, 2000 at our present time, and a relatively close galaxy (outside our Local Group) with a value of N1 = 1990, that galaxy would have a redshift, z = 0.00502. This means we can determine the number of integer changes in ho since the moment light was emitted by the distant galaxy. But it was shown in Reviewing the Zero Point Energy and Quantized Redshifts and the Zero Point Energy that (1 + z) was inversely proportional to the strength of the ZPE, U. Our conclusion is that
and that this accords with what has been published in the papers linked above. Therefore, it can be stated that, while the concept was right in Quantized Redshifts and the Zero Point Energy, the mathematical approach to the concept was in error. That incorrect approach has thus been corrected here. We thus thank the reviewer for pointing out this problem. |